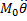
1. A  long cylindrical rod of radius R has a magnetization in the azimuthal direction.  There are no free currents. Find the magnetic field both inside and outside the cylinder due to magnetization.

The bound volume current is zero since the magnetization  has constant magnitude. The surface current density is 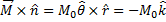. The magnetic field  inside is zero as there  is no volume current inside. The current outside is given by

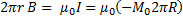
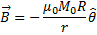
2. In the preceding problem, take the magnetization to be given by 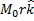where r is the distance from the cylindrical axis. Find the magnetic field both inside and outside the cylinder due to magnetization.

Bound current densities are as follows 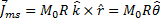 and

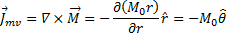
By symmetry the fields at The total current enclosed  within a length L of the cylinder  is 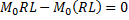. Thus the magnetic field outside is zero.

Since the current is in azimuthal direction, in order to calculate the magnetic field, we  need to take an Amperian loop which is in the form of a rectangle of height L  whose one side is at a distance r from the axis and the other parallel side is outside.  Since the field outside is zero, we have,

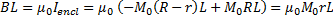
which gives  .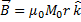

3. Repeat Problem 2 by calculating the H field and adding the magnetization contribution.

Since there are no free currents, H=0. Thus 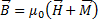.Since the magnetization is zero outside, the field is zero. Inside, the field is equal to .

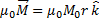4. A magnetized material is in the shape of a cube of side a  as shown. The magnetization is directed along the z direction and varies linearly  from its value zero on  its surface on the y-z plane to a value 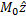 on the plane 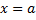. Calculate the bound currents both on the surface and inside the material.

Since the magnetization varies linearly  with x, the magnetization is given by . The bound current density in the volume is . The surface bound currents are as follows. On the face x=0, there is no bound current because M=0 on that face. On the face , the normal direction is  so that the bound current density is . On the top face, the normal direction is  and the magnetization is  so that the bound current density is  zero. We have a contribution from the  face for which the normal is directed along  and the  bound current density is , the current on the opposite face is oppositely directed.